. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
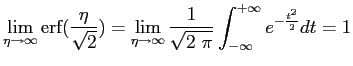
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
http://www.see.ed.ac.uk/ johnc/teaching/fluidmechanics4/2003-04/fluids11/momentum.htmlhttp://www.see.ed.ac.uk/ johnc/teaching/fluidmechanics4/2003-04/fluids11/momentum.html
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
http://planetmath.org/encyclopedia/DifferentiationUnderIntegralSign.htmlhttp://planetmath.org/encyclopedia/DifferentiationUnderIntegralSign.html
http://www.math.ualberta.ca/ etalvila/papers/difffinal.pdfhttp://www.math.ualberta.ca/ etalvila/papers/difffinal.pdf.
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
http://www.see.ed.ac.uk/johnc/teaching/fluidmechanics4/2003-04/fluids9/2-dboundary.htmlwww.see.ed.ac.uk/~johnc/teaching/fluidmechanics4/2003-04/fluids9/2-dboundary.html
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
http://www.dpa.unina.it/coiro/esercitazioni/download/runge-kutta.pdfhttp://www.dpa.unina.it/coiro/esercitazioni/download/runge-kutta.pdf
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
o anche [6] per una trattazione sui metodi numerici per la soluzione delle ODE.
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
http://www.ecs.fullerton.edu/ mathews/n2003/rungekutta/RungeKuttaProof.pdfhttp://www.ecs.fullerton.edu/ mathews/n2003/rungekutta/RungeKuttaProof.pdf relativamente alla scelta dei coefficienti.
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .